Right Triangle Calculator
Right triangle calculator calculates the side length, angle, area, or perimeter from another known side or angle for a right triangle.
Right Angle Triangle Calculator |
|
| Known | |
| Leg a | |
| Leg b | |
| Hypotenuse c | |
| Angle X | ° (in Degree) |
| Angle Y | ° (in Degree) |
| Results | |
| Hypotenuse c | 43.01 |
| Leg a | |
| Leg b | |
| Angle X | 35.54° |
| Angle Y | 54.46° |
| Area | 437.5 |
| Perimeter | 103.01 |
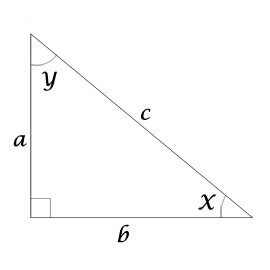
A right triangle is a triangle with one angle that is 90°(also called the right angle). The two sides of the right angle are the leg, and the opposite side of
the right angle is the hypotenuse.
The area formula for a right triangle is:
A = (a x b)/2
(a and b are both legs for the right triangle)
The perimeter formula for a right triangle is adding all the sides together.
P=a+b+c.
Find hypotenuse of a right triangle
To find hypotenuse c by giving two legs:
c=√(a2+b2)
To find hypotenuse by giving a leg and an angle, we need to apply the law of sin formula
which is:
a⁄sin(X)=b⁄sin(Y)=c⁄sin(90°)
(sin90°=1)
Therefore
c= a⁄sin(X) OR
c=b⁄sin(Y)
Find the side of a right triangle
To find a leg by known leg and hypotenuse
a = c2-b2
OR
b =c2-a2
By known hypotenuse and an angle.
a=c x sin(X).
b=c x sin(Y)
Find the angle of a right triangle
There are multiway to find an angle of a right triangle.
by known two other sides (either legs or hypotenuse).
To find angle X:
X=sin-1(a/c)
X=cos-1(b/c)
X=tan-1(a/b)
X=cot-1(b/a)
To find angle Y:
Y=sin-1(b/c)
Y=cos-1(a/c)
Y=tan-1(b/a)
Y=cot-1(a/b)
By known the other angle:
X=90°-Y OR Y=90°-X
(because a triangle is 180° for 3 angles altogether, the right angle is 90°, and the other two angles are 180-90=90°)
- 30 60 90 Triangle Calculator
- 3d Distance Calculator
- 45 45 90 Triangle Calculator
- Absolute Change Calculator
- Absolute Uncertainty Calculator
- Absolute Value Calculator
- Acceleration Calculator
- Annulus Calculator
- Arc Length Calculator
- Area Of Ellipse Calculator
- Area Of Parallelogram Calculator
- Area Of Semicircle Calculator
- Arithmetic Sequence Calculator
- Base Converter Calculator
- Binary To Decimal Calculator
- Binary To Hex Calculator
- Binary To Octal Calculator
- Binary Calculator
- Binomial Probability Calculator
- Birthday Paradox Calculator
- Bradford Factor Calculator
- Central Angle Calculator
- Central Limit Theorem Calculator
- Circular Permutation Calculator
- Circumference Of Oval Calculator
- Class Width Calculator
- Clock Angle Calculator
- Coin Flip Probability Calculator
- Combination With Replacement Calculator
- Complementary And Supplementary Angles Calculator
- Confidence Interval Calculator
- Consecutive Integers Calculator
- Constant Of Proportionality Calculator
- Covariance Calculator
- Cross Multiply Calculator
- Cube Surface Area Calculator
- Cube Volume Calculator
- Cylinder Surface Area Calculator
- Cylinder Volume Calculator
- Decimal Random Number Generator Calculator
- Decimal To Binary Calculator
- Decimal To Hex Calculator
- Decimal To Inch Calculator
- Decimal To Octal Calculator
- Digital Root Calculator
- Distance Formula Calculator
- Dividing Exponents Calculator
- Division Calculator
- Double Factorial Calculator
- Empirical Rule Calculator
- Equilateral Triangle Calculator
- Find The Perimeter Of The Polygon Calculator
- Fourth Roots Calculator
- Fractional Exponents Calculator
- Gcf Calculator
- Geometric Distribution Calculator
- Geometric Mean Calculator
- Geometric Sequence Calculator
- Golden Ratio Calculator
- Greater Than Or Less Than Calculator
- Height Of Trapezoid Calculator
- Herons Formula Calculator
- Hex To Binary Calculator
- Hex To Decimal Calculator
- Hex To Octal Calculator
- Hypergeometric Distribution Calculator
- Hypotenuse Calculator
- Inch Fraction To Mm Calculator
- Inches To Decimal Calculator
- Law Of Sines Calculator
- Lcm Calculator
- Linear Equation Calculator
- Log Base 10 Calculator
- Margin Of Error Calculator
- Mm To Inch Fraction Calculator
- Multiplying Exponents Calculator
- Natural Log Calculator
- Numberbaseconverter Calculator
- Octal To Binary Calculator
- Octal To Decimal Calculator
- Octal To Hex Calculator
- One Million In Numbers Calculator
- Perfect Cube Calculator
- Perfect Square Calculator
- Perimeter Of Parallelogram Calculator
- Perimeter Of Semicircle Calculator
- Permutation With Repetition Calculator
- Pipe Volume Calculator
- Population Density Calculator
- Population Growth Calculator
- Power Mod Calculator
- Powers Of Fraction Calculator
- Prime Number Calculator
- Probability Of 2 Events Calculator
- Probability Of 3 Events Calculator
- Pyramid Volume Calculator
- Quadratic Equation Calculator
- Raw Score Calculator
- Reciprocal Calculator
- Rectangular Surface Area Calculator
- Rectangular Volume Calculator
- Reference Angle Calculator
- Remainder Calculator
- Right Triangle Calculator
- Roman Numbers 1 To 100 Calculator
- Rounding Calculator
- Scientific Notation Calculator
- Sector Area Calculator
- Sgpa To Percentage Calculator
- Sig Fig Calculator
- Slope Percentage Calculator
- Sphere Surface Area Calculator
- Square Foot Calculator
- Square In A Circle Calculator
- Square Pyramid Surface Area Calculator
- Stadium Calculator
- Standard Error Calculator
- Statistics Calculator
- Sum Of Factors Calculator
- Sum Calculator
- Surface Area Calculator
- T Value Calculator
- Tangent Of A Circle Calculator
- Time Percentage Calculator
- Trapezoid Area Calculator
- Triangle Inequality Theorem Calculator
- Triangular Volume Calculator
- Triple Factorial Calculator
- Volume Of A Cone Calculator
- Volume Of A Sphere Calculator
- Weighted Average Calculator
- Weighted Mean Calculator
- What Plus What Equals Calculator
- Xis What Percent Ofy Calculator
Terms | Privacy | Disclaimer | Contact
©2025 bCalculator